At first glance the 1680 digit number
111111111111111111111111111111111111111111
111111111111111111111111111111111111111111
111111999999911111111111111111111111111111
111111111111111999999999999999999999991111
111111111111111111111111111111119999999999
999999999999999999111111111111111111111111
111111111199999999995555555599999999991111
111111111111111111111111199999999999999555
555555559999999911111111111111111111111999
999999555555555555555555555559999999111111
111111111111199999955555555555555999995555
555555599999911111111111111199999955555555
555555555555599999555555559999911111111111
111999999999999995555555555555555599999555
555999991111111111199999999999999555555555
555555555555997995555599991111111111999999
999999995555555555555555555555597799555559
991111111119999999999999555555599955555555
599795591199999559991111111199991111999995
555999995555555555591179999111995559999111
111991111119999955999999955555555555991197
799999555599999111111111111199999999999995
555555555555599991119799559995999911111111
111199999999999955555555555555591191199991
919999999911111111111999999999999955999999
555555559191119111999999999911111111111999
999999999999999999995555712499999999999999
999911111111119999999199999999999999999955
555555555559999999999911111111119999911199
999999999999999999955555555999991119999111
111111199999111199991111111111999999999999
999999111119999111111111199911111999111111
111111111999999999999111111119991111111111
199111111911111111111111111111111111111111
111199911111111111991111111111111111111111
111111111111111111111199111111111111911111
111111111111111111111111111111111111111111
111111111111111111111111111111111111111111
111111111111111111111111111111111111111111
111111111111111111111111111111111111111111
would not appear to be remarkable, other than in the preponderance of 1’s and 9’s that comprise its digits. However, there are two interesting observations about this number. First, it is prime. The only integers that divide evenly into it are 1 and itself. Second, if the digits are arranged in a rectangular fashion, 60 digits to a row, we see why it has been dubbed the “River Hawk Prime.”

 This fall we are welcoming Dr. Sedi Bartz to the Department of Mathematical Sciences. Dr. Bartz’ research focuses on topics of nonlinear analysis and variational analysis. He develops refinements of abstract convex analysis, and in turn, transforms his refinements into a unifying language for phenomena in variational analysis which used to be considered quite apart. Dr. Bartz is also a specialist in classical convex analysis and monotone operator theory, theories which are among the most popular tools of modern optimization.
This fall we are welcoming Dr. Sedi Bartz to the Department of Mathematical Sciences. Dr. Bartz’ research focuses on topics of nonlinear analysis and variational analysis. He develops refinements of abstract convex analysis, and in turn, transforms his refinements into a unifying language for phenomena in variational analysis which used to be considered quite apart. Dr. Bartz is also a specialist in classical convex analysis and monotone operator theory, theories which are among the most popular tools of modern optimization.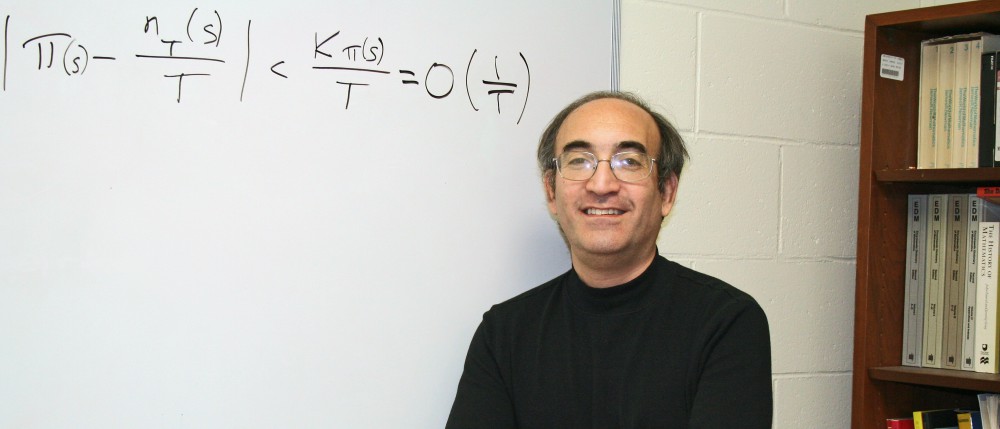
 Dr Guha’s research interest include Bayesian Modeling, Inverse problems, Uncertainty Quantification, High-dimensional Problems and Graphical Modeling.
Dr Guha’s research interest include Bayesian Modeling, Inverse problems, Uncertainty Quantification, High-dimensional Problems and Graphical Modeling.