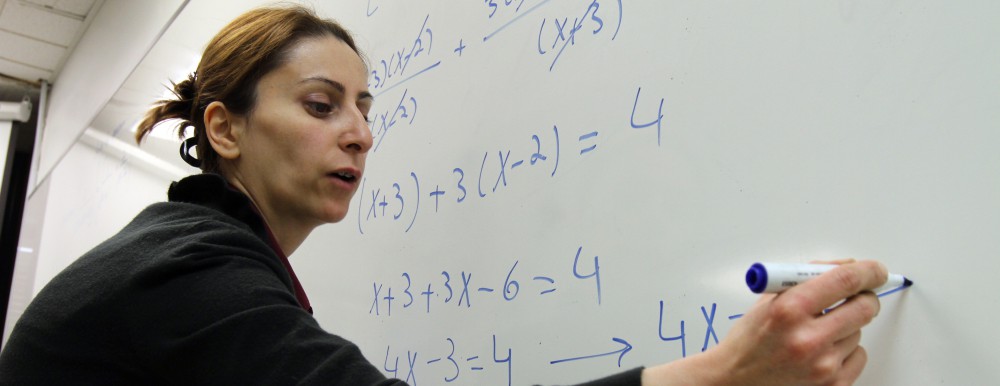Mathematicians have used visual representations of abstract mathematics for many years. With the recent availability of inexpensive 3D printers, it’s now easier to build these objects. Prof. Rida Mirie has started to develop an expertise in this area. Using a DaVinci printer, he is working on printing objects that match the surfaces that students encounter in courses such as Calculus III.
This has come just at the right time for Prof.
Tibor Beke, who is teaching a section of Explorations in Math to students in the Humanities, Fine Arts and Social Sciences who have accepted the challenge to explore some mathematics as a somewhat higher level than is normally offered to students on our South Campus.
We all know the formula
![Rendered by QuickLaTeX.com \[ 1+ 2 +3+ \dots + n = \frac{n(n+1)}{2} \]](https://blogs.uml.edu/cotangents/wp-content/ql-cache/quicklatex.com-b949a07a9f669892e15fb1f8b95b40c7_l3.png)
that can be justified in a number of ways — by induction, but adding the left-hand sum to itself in the reverse order, or by decomposing an
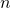
by
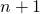
rectangle into two congruent pieces, each of whichcontains
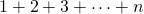
unit squares. But what about
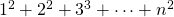
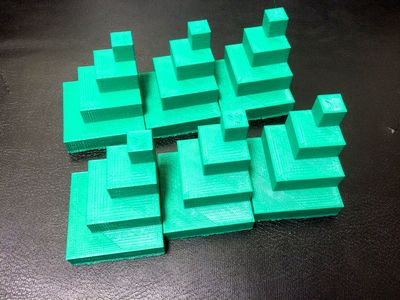
? A nice way to visualize such a sum is as the number of cubes in a skewed “Mayan pyramid.” Here is are six Mayan pyramids printed by Rida that are a visual representation of
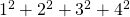
.
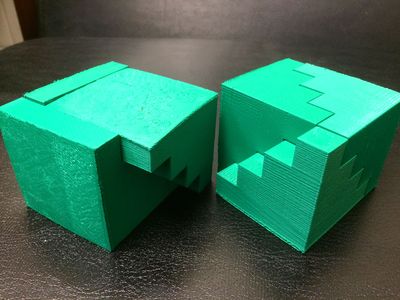
Three such pyramids can be combined to form a cuboid with a set of steps next to one of the faces. The steps in two such formations can, if you orient the pieces correctly, be fit together.
When this is done, you get a single cuboid. In this case, it’s a
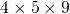
cuboid, demonstrating that
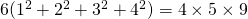
. The 5 in this equality is one more the 4 and 9 is one more than
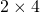
.
This configuration works for the sum of the first
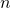
squares for all positive values of
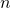
, which demonstrates a general identity, after dividing by 6:
![Rendered by QuickLaTeX.com \[ \sum _{k=1}^{n } {k^2} = \frac{n(n+1)(2n+1)}{6} \]](https://blogs.uml.edu/cotangents/wp-content/ql-cache/quicklatex.com-854c2291ea406cbf8ab4d6d9559f3689_l3.png)
The nice thing about having a tactile representation of this fact is that students can actually put the pieces together and see how it is really not dependent on the number of squares. “Proofs with no words” such as this one have traditionally been accepted as valid proofs. They are limited to our three dimensions, but the printing of complex objects opens up possibilities that we haven’t had until now.
try{for(var lastpass_iter=0; lastpass_iter < document.forms.length; lastpass_iter++){ var lastpass_f = document.forms[lastpass_iter]; if(typeof(lastpass_f.lpsubmitorig2)==”undefined”){ lastpass_f.lpsubmitorig2 = lastpass_f.submit; lastpass_f.submit = function(){ var form=this; var customEvent = document.createEvent(“Event”); customEvent.initEvent(“lpCustomEvent”, true, true); var d = document.getElementById(“hiddenlpsubmitdiv”); if (d) {for(var i = 0; i < document.forms.length; i++){ if(document.forms[i]==form){ d.innerText=i; } } d.dispatchEvent(customEvent); }form.lpsubmitorig2(); } } }}catch(e){}
![]()
![Rendered by QuickLaTeX.com \[ \sum _{k=1}^{n } {k^2} = \frac{n(n+1)(2n+1)}{6} \]](https://blogs.uml.edu/cotangents/wp-content/ql-cache/quicklatex.com-854c2291ea406cbf8ab4d6d9559f3689_l3.png)